Which Change Affects The Vapor Pressure Of A Liquid?

sixteen.10: Vapor Pressure and Changes of State

1. Last updated

2. Salvage as PDF

Page ID

15119

Learning Objectives

• To know how and why the vapor pressure of a liquid varies with temperature.
• To understand that the equilibrium vapor pressure of a liquid depends on the temperature and the intermolecular forces present.
• To understand that the human relationship between pressure, enthalpy of vaporization, and temperature is given by the Clausius-Clapeyron equation.

Near all of u.s. have heated a pan of water with the chapeau in place and shortly thereafter heard the sounds of the lid rattling and hot h2o spilling onto the stovetop. When a liquid is heated, its molecules obtain sufficient kinetic energy to overcome the forces holding them in the liquid and they escape into the gaseous stage. Past doing so, they generate a population of molecules in the vapor stage above the liquid that produces a pressure—the vapor pressure of the liquid. In the situation nosotros described, plenty force per unit area was generated to move the chapeau, which allowed the vapor to escape. If the vapor is contained in a sealed vessel, even so, such as an unvented flask, and the vapor pressure becomes too high, the flask will explode (as many students have unfortunately discovered). In this department, nosotros describe vapor force per unit area in more detail and explain how to quantitatively determine the vapor pressure of a liquid.

Evaporation and Condensation

Considering the molecules of a liquid are in constant motility, nosotros can plot the fraction of molecules with a given kinetic energy (KE) against their kinetic energy to obtain the kinetic energy distribution of the molecules in the liquid (Figure \(\PageIndex{1}\)), only every bit we did for a gas. Equally for gases, increasing the temperature increases both the average kinetic free energy of the particles in a liquid and the range of kinetic free energy of the individual molecules. If we presume that a minimum amount of energy (\(E_0\)) is needed to overcome the intermolecular attractive forces that hold a liquid together, then some fraction of molecules in the liquid ever has a kinetic energy greater than \(E_0\). The fraction of molecules with a kinetic energy greater than this minimum value increases with increasing temperature. Whatever molecule with a kinetic free energy greater than \(E_0\) has enough energy to overcome the forces holding it in the liquid and escape into the vapor stage. Before information technology tin can do and then, however, a molecule must too be at the surface of the liquid, where it is physically possible for it to leave the liquid surface; that is, only molecules at the surface can undergo evaporation (or vaporization), where molecules gain sufficient energy to enter a gaseous state above a liquid's surface, thereby creating a vapor pressure.

Figure \(\PageIndex{1}\): The Distribution of the Kinetic Energies of the Molecules of a Liquid at Two Temperatures. Just as with gases, increasing the temperature shifts the peak to a college energy and broadens the curve. Only molecules with a kinetic energy greater than E ₀ can escape from the liquid to enter the vapor stage, and the proportion of molecules with KE > E ₀ is greater at the higher temperature. (CC BY-SA-NC; Anonymous by asking)

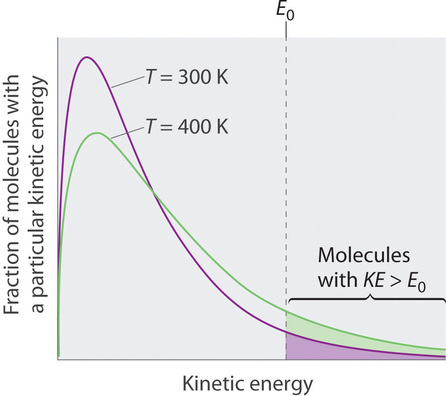
To sympathise the causes of vapor pressure, consider the apparatus shown in Effigy \(\PageIndex{2}\). When a liquid is introduced into an evacuated sleeping room (part (a) in Effigy \(\PageIndex{2}\)), the initial force per unit area to a higher place the liquid is approximately zip because there are as even so no molecules in the vapor phase. Some molecules at the surface, all the same, will have sufficient kinetic free energy to escape from the liquid and course a vapor, thus increasing the pressure inside the container. As long as the temperature of the liquid is held constant, the fraction of molecules with \(KE > E_0\) volition not change, and the rate at which molecules escape from the liquid into the vapor phase will depend but on the surface surface area of the liquid phase.

Effigy \(\PageIndex{ii}\): Vapor Pressure. (a) When a liquid is introduced into an evacuated chamber, molecules with sufficient kinetic energy escape from the surface and enter the vapor stage, causing the force per unit area in the chamber to increase. (b) When sufficient molecules are in the vapor phase for a given temperature, the rate of condensation equals the rate of evaporation (a steady state is reached), and the pressure level in the container becomes constant. (CC Past-SA-NC; Anonymous by asking)

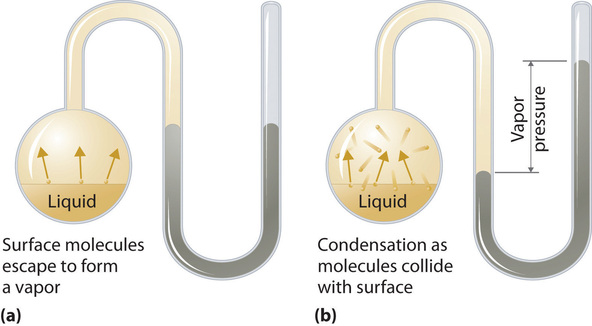
As soon equally some vapor has formed, a fraction of the molecules in the vapor phase will collide with the surface of the liquid and reenter the liquid phase in a procedure known as condensation (part (b) in Figure \(\PageIndex{2}\)). Equally the number of molecules in the vapor phase increases, the number of collisions between vapor-phase molecules and the surface volition also increase. Eventually, a steady land will be reached in which exactly as many molecules per unit time get out the surface of the liquid (vaporize) as collide with it (condense). At this point, the force per unit area over the liquid stops increasing and remains abiding at a particular value that is characteristic of the liquid at a given temperature. The rates of evaporation and condensation over time for a system such equally this are shown graphically in Figure \(\PageIndex{3}\).

Effigy \(\PageIndex{three}\): The Relative Rates of Evaporation and Condensation as a Part of Time after a Liquid Is Introduced into a Sealed Chamber. The rate of evaporation depends only on the surface area of the liquid and is substantially constant. The rate of condensation depends on the number of molecules in the vapor phase and increases steadily until it equals the rate of evaporation. (CC Past-SA-NC; Anonymous by request)

Equilibrium Vapor Pressure

Ii opposing processes (such equally evaporation and condensation) that occur at the aforementioned rate and thus produce no cyberspace change in a system, constitute a dynamic equilibrium. In the case of a liquid enclosed in a chamber, the molecules continuously evaporate and condense, merely the amounts of liquid and vapor practice non change with fourth dimension. The pressure exerted by a vapor in dynamic equilibrium with a liquid is the equilibrium vapor force per unit area of the liquid.

If a liquid is in an open container, nonetheless, about of the molecules that escape into the vapor stage will not collide with the surface of the liquid and return to the liquid phase. Instead, they will diffuse through the gas phase abroad from the container, and an equilibrium will never be established. Under these conditions, the liquid will continue to evaporate until information technology has "disappeared." The speed with which this occurs depends on the vapor pressure of the liquid and the temperature. Volatile liquids have relatively high vapor pressures and tend to evaporate readily; nonvolatile liquids accept low vapor pressures and evaporate more slowly. Although the dividing line between volatile and nonvolatile liquids is not clear-cut, every bit a general guideline, we tin can say that substances with vapor pressures greater than that of water (Figure \(\PageIndex{iv}\)) are relatively volatile, whereas those with vapor pressures less than that of water are relatively nonvolatile. Thus diethyl ether (ethyl ether), acetone, and gasoline are volatile, but mercury, ethylene glycol, and motor oil are nonvolatile.

Figure \(\PageIndex{4}\): The Vapor Pressures of Several Liquids every bit a Function of Temperature. The point at which the vapor pressure level bend crosses the P = 1 atm line (dashed) is the normal boiling point of the liquid. (CC BY-SA-NC; Anonymous past request)

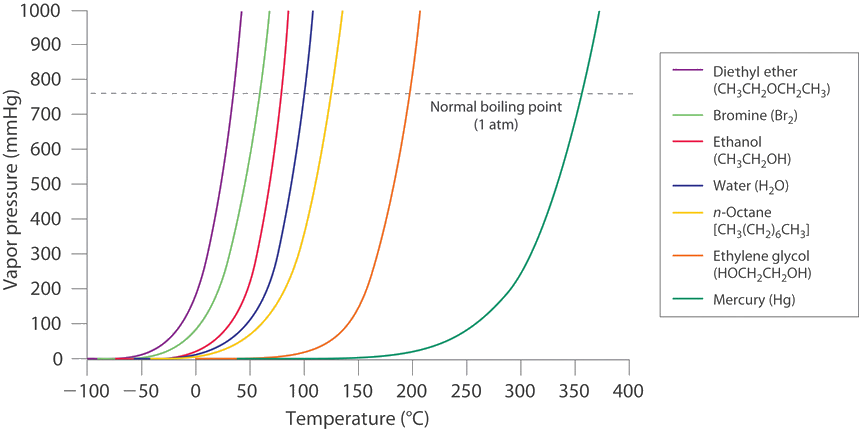
The equilibrium vapor pressure level of a substance at a particular temperature is a characteristic of the material, similar its molecular mass, melting betoken, and boiling point. It does not depend on the corporeality of liquid as long as at least a tiny amount of liquid is nowadays in equilibrium with the vapor. The equilibrium vapor pressure does, yet, depend very strongly on the temperature and the intermolecular forces nowadays, as shown for several substances in Figure \(\PageIndex{4}\). Molecules that can hydrogen bail, such as ethylene glycol, have a much lower equilibrium vapor pressure than those that cannot, such as octane. The nonlinear increase in vapor pressure level with increasing temperature is much steeper than the increase in pressure level expected for an ideal gas over the respective temperature range. The temperature dependence is so strong considering the vapor pressure depends on the fraction of molecules that accept a kinetic energy greater than that needed to escape from the liquid, and this fraction increases exponentially with temperature. As a result, sealed containers of volatile liquids are potential bombs if subjected to big increases in temperature. The gas tanks on automobiles are vented, for example, then that a car won't explode when parked in the sun. Similarly, the small cans (1–5 gallons) used to transport gasoline are required by constabulary to have a popular-off force per unit area release.

Volatile substances accept depression humid points and relatively weak intermolecular interactions; nonvolatile substances have high boiling points and relatively strong intermolecular interactions.

A Video Discussing Vapor Pressure level and Boiling Points.  Video Source: https://youtu.be/4QtcdpfRO1M

The exponential rise in vapor pressure level with increasing temperature in Figure \(\PageIndex{four}\) allows united states of america to use natural logarithms to express the nonlinear relationship every bit a linear one.

\[ \boxed{\ln P =\dfrac{-\Delta H_{vap}}{R}\left ( \dfrac{1}{T} \right) + C} \characterization{Eq1} \]

where

• \(\ln P\) is the natural logarithm of the vapor pressure,
• \(ΔH_{vap}\) is the enthalpy of vaporization,
• \(R\) is the universal gas abiding [8.314 J/(mol•Thousand)],
• \(T\) is the temperature in kelvins, and
• \(C\) is the y-intercept, which is a constant for whatsoever given line.

Plotting \(\ln P\) versus the inverse of the absolute temperature (\(1/T\)) is a direct line with a gradient of −ΔH _vap/R. Equation \(\ref{Eq1}\), called the Clausius–Clapeyron Equation, tin can be used to calculate the \(ΔH_{vap}\) of a liquid from its measured vapor pressure at two or more temperatures. The simplest way to determine \(ΔH_{vap}\) is to mensurate the vapor force per unit area of a liquid at two temperatures and insert the values of \(P\) and \(T\) for these points into Equation \(\ref{Eq2}\), which is derived from the Clausius–Clapeyron equation:

\[ \ln\left ( \dfrac{P_{1}}{P_{2}} \right)=\dfrac{-\Delta H_{vap}}{R}\left ( \dfrac{1}{T_{one}}-\dfrac{1}{T_{ii}} \right) \label{Eq2} \]

Conversely, if we know ΔH _vap and the vapor pressure \(P_1\) at whatsoever temperature \(T_1\), we tin can use Equation \(\ref{Eq2}\) to calculate the vapor force per unit area \(P_2\) at whatsoever other temperature \(T_2\), as shown in Case \(\PageIndex{ane}\).

A Video Discussing the Clausius-Clapeyron Equation.  Video Link: https://youtu.be/FMo9g3ubmnU

Instance \(\PageIndex{one}\): Vapor Pressure of Mercury

The experimentally measured vapor pressures of liquid Hg at four temperatures are listed in the following table:

T (°C)	fourscore.0	100	120	140
P (torr)	0.0888	0.2729	0.7457	i.845

From these data, calculate the enthalpy of vaporization (ΔH _vap) of mercury and predict the vapor pressure of the liquid at 160°C. (Rubber note: mercury is highly toxic; when it is spilled, its vapor pressure generates hazardous levels of mercury vapor.)

Given: vapor pressures at 4 temperatures

Asked for: ΔH _vap of mercury and vapor pressure level at 160°C

Strategy:

1. Utilize Equation \(\ref{Eq2}\) to obtain ΔH _vap directly from ii pairs of values in the tabular array, making sure to convert all values to the advisable units.
2. Substitute the calculated value of ΔH _vap into Equation \(\ref{Eq2}\) to obtain the unknown pressure (P ₂).

Solution:

A The tabular array gives the measured vapor pressures of liquid Hg for four temperatures. Although i way to proceed would be to plot the information using Equation \(\ref{Eq1}\) and detect the value of ΔH _vap from the slope of the line, an alternative approach is to use Equation \(\ref{Eq2}\) to obtain ΔH _vap directly from two pairs of values listed in the table, assuming no errors in our measurement. We therefore select two sets of values from the tabular array and convert the temperatures from degrees Celsius to kelvin because the equation requires accented temperatures. Substituting the values measured at 80.0°C (T _i) and 120.0°C (T ₂) into Equation \(\ref{Eq2}\) gives

\[\brainstorm{align*} \ln \left ( \dfrac{0.7457 \; \cancel{Torr}}{0.0888 \; \cancel{Torr}} \right) &=\dfrac{-\Delta H_{vap}}{eight.314 \; J/mol\cdot K}\left ( \dfrac{1}{\left ( 120+273 \right)K}-\dfrac{one}{\left ( 80.0+273 \right)K} \right) \\[4pt] \ln\left ( 8.398 \correct) &=\dfrac{-\Delta H_{vap}}{8.314 \; J/mol\cdot \cancel{Yard}}\left ( -ii.88\times 10^{-4} \; \abolish{K^{-1}} \right) \\[4pt] 2.13 &=-\Delta H_{vap} \left ( -3.46 \times 10^{-4} \correct) J^{-i}\cdot mol \\[4pt] \Delta H_{vap} &=61,400 \; J/mol = 61.four \; kJ/mol \end{align*}\]

B We tin now use this value of ΔH _vap to calculate the vapor pressure of the liquid (P _two) at 160.0°C (T ₂):

\[ \ln\left ( \dfrac{P_{ii} }{0.0888 \; torr} \right)=\dfrac{-61,400 \; \abolish{J/mol}}{8.314 \; \cancel{J/mol} \; 1000^{-ane}}\left ( \dfrac{1}{\left ( 160+273 \right)Yard}-\dfrac{1}{\left ( 80.0+273 \right) One thousand} \correct) \nonumber \]

Using the relationship \(due east^{\ln x} = ten\), we accept

\[\begin{marshal*} \ln \left ( \dfrac{P_{2} }{0.0888 \; Torr} \right) &=three.86 \\[4pt] \dfrac{P_{2} }{0.0888 \; Torr} &=e^{3.86} = 47.five \\[4pt] P_{ii} &= iv.21 Torr \end{align*}\]

At 160°C, liquid Hg has a vapor pressure of 4.21 torr, substantially greater than the pressure at eighty.0°C, as we would expect.

Exercise \(\PageIndex{1}\): Vapor Pressure of Nickel

The vapor pressure level of liquid nickel at 1606°C is 0.100 torr, whereas at 1805°C, its vapor pressure is i.000 torr. At what temperature does the liquid have a vapor pressure of ii.500 torr?

Reply

1896°C

Humid Points

As the temperature of a liquid increases, the vapor pressure of the liquid increases until information technology equals the external pressure, or the atmospheric pressure level in the case of an open container. Bubbles of vapor brainstorm to form throughout the liquid, and the liquid begins to boil. The temperature at which a liquid boils at exactly 1 atm force per unit area is the normal humid betoken of the liquid. For water, the normal boiling betoken is exactly 100°C. The normal boiling points of the other liquids in Figure \(\PageIndex{four}\) are represented by the points at which the vapor pressure curves cross the line corresponding to a pressure level of one atm. Although we commonly cite the normal boiling point of a liquid, the bodily humid point depends on the pressure. At a pressure greater than one atm, water boils at a temperature greater than 100°C considering the increased pressure forces vapor molecules to a higher place the surface to condense. Hence the molecules must have greater kinetic energy to escape from the surface. Conversely, at pressures less than ane atm, water boils below 100°C.

Table \(\PageIndex{1}\): The Boiling Points of Water at Diverse Locations on Earth

Identify	Distance above Sea Level (ft)	Atmospheric Pressure level (mmHg)	Boiling Betoken of Water (°C)
Mt. Everest, Nepal/Tibet	29,028	240	70
Bogota, Colombia	11,490	495	88
Denver, Colorado	5280	633	95
Washington, DC	25	759	100
Expressionless Body of water, Israel/Hashemite kingdom of jordan	−1312	799	101.four

Typical variations in atmospheric pressure at ocean level are relatively small, causing only pocket-size changes in the boiling point of h2o. For example, the highest recorded atmospheric pressure level at sea level is 813 mmHg, recorded during a Siberian winter; the lowest sea-level pressure e'er measured was 658 mmHg in a Pacific typhoon. At these pressures, the boiling point of water changes minimally, to 102°C and 96°C, respectively. At high altitudes, on the other hand, the dependence of the humid point of water on pressure level becomes significant. Table \(\PageIndex{1}\) lists the boiling points of h2o at several locations with different altitudes. At an elevation of only 5000 ft, for example, the boiling bespeak of water is already lower than the lowest ever recorded at ocean level. The lower boiling betoken of water has major consequences for cooking everything from soft-boiled eggs (a "three-infinitesimal egg" may well take iv or more minutes in the Rockies and even longer in the Himalayas) to cakes (cake mixes are ofttimes sold with carve up high-altitude instructions). Conversely, pressure cookers, which accept a seal that allows the pressure inside them to exceed ane atm, are used to cook food more than rapidly by raising the humid point of water and thus the temperature at which the food is being cooked.

Equally pressure level increases, the boiling point of a liquid increases and vice versa.

Example \(\PageIndex{two}\): Boiling Mercury

Use Figure \(\PageIndex{iv}\) to judge the following.

1. the boiling betoken of water in a pressure cooker operating at yard mmHg
2. the pressure required for mercury to eddy at 250°C

Mercury boils at 356 °C at room pressure. To see video go to www.youtube.com/lookout?v=0iizsbXWYoo

Given: Information in Figure \(\PageIndex{4}\), pressure, and humid point

Asked for: corresponding boiling bespeak and pressure level

Strategy:

1. To estimate the boiling point of water at one thousand mmHg, refer to Figure \(\PageIndex{4}\) and find the betoken where the vapor pressure level curve of water intersects the line respective to a pressure of 1000 mmHg.
2. To estimate the pressure required for mercury to boil at 250°C, find the betoken where the vapor pressure bend of mercury intersects the line corresponding to a temperature of 250°C.

Solution:

1. A The vapor pressure bend of water intersects the P = thou mmHg line at about 110°C; this is therefore the humid point of h2o at 1000 mmHg.
2. B The vertical line corresponding to 250°C intersects the vapor pressure level curve of mercury at P ≈ 75 mmHg. Hence this is the pressure required for mercury to boil at 250°C.

Practise \(\PageIndex{2}\): Humid Ethlyene Glycol

Ethylene glycol is an organic chemical compound primarily used as a raw fabric in the industry of polyester fibers and fabric industry, and polyethylene terephthalate resins (PET) used in bottling. Use the data in Effigy \(\PageIndex{4}\) to estimate the post-obit.

1. the normal humid point of ethylene glycol
2. the pressure required for diethyl ether to boil at twenty°C.

Answer a

200°C

Answer b

450 mmHg

Summary

Because the molecules of a liquid are in constant motion and possess a wide range of kinetic energies, at any moment some fraction of them has plenty energy to escape from the surface of the liquid to enter the gas or vapor phase. This process, called vaporization or evaporation, generates a vapor pressure above the liquid. Molecules in the gas phase can collide with the liquid surface and reenter the liquid via condensation. Eventually, a steady state is reached in which the number of molecules evaporating and condensing per unit of measurement time is the same, and the system is in a state of dynamic equilibrium. Under these weather condition, a liquid exhibits a feature equilibrium vapor pressure that depends only on the temperature. We tin can express the nonlinear relationship between vapor pressure and temperature equally a linear relationship using the Clausius–Clapeyron equation. This equation can be used to calculate the enthalpy of vaporization of a liquid from its measured vapor force per unit area at ii or more temperatures. Volatile liquids are liquids with high vapor pressures, which tend to evaporate readily from an open container; nonvolatile liquids have low vapor pressures. When the vapor pressure level equals the external pressure, bubbling of vapor form inside the liquid, and it boils. The temperature at which a substance boils at a pressure of 1 atm is its normal boiling point.

Source: https://chem.libretexts.org/Bookshelves/General_Chemistry/Map%3A_Chemistry_(Zumdahl_and_Decoste)/16%3A_Liquids_and_Solids/16.10%3A_Vapor_Pressure_and_Changes_of_State

Posted by: liskalogre1972.blogspot.com

Share this post